Physics 101 - Homework # 1 Solutions
Chapter 1
Problem 3)
- Cube root = (1021)1/3 = 1021/3 =
107
Square = (107)2 =
1014
Problem 8)
Remember: to convert units, just multiply by "1"...
- Price = (1.90 DM/L)($1/1.7 DM)(3.8 L/gal) =
$4.25/gal
(and you thought gas was expensive here!)
Problem 10)
- This is a unit conversion problem [note the typo in the question; dimensional
analysis tells us that the units of G to convert to must be
cm3s-2g-1 not cm3s2g-1,
since the dimensions are L3T-2M-1 ]. Convert
by multiplying successively by "1":
-
G = 6.67 x 10-11 m3s-2kg-1
(100 cm/1 m)3(kg/1000 g)
- = 6.67 x 10-8
cm3s-2g-1
- (note that the factor of 100 from m to cm gets cubed... we will discuss G
further later in the course... )
Problem 23)
- Dimensional Analysis: For L = h/mec we can write the
dimensions as
- [L] = [h][me]-1[c]-1
= [h][M]-1([L][T]-1)-1
- thus
- [h] = [L][M] [L][T]-1 = [ML2T-1]
- (thus in SI units h would be in kg m2/s;
h is known as Planck's constant, and is central in Quantum mechanics...)
Chapter 2
Problem 1)
- The net displacement is the sum of each individual displacement:
- = + 32 cm - 27 cm - 23 cm + 39 cm = +21 cm
- Since the initial position in this case was the origin, the
grasshopper is 21 cm from the origin in the positive direction.
Problem 6)
- This can be solved graphically using a plot of distance
vs. time
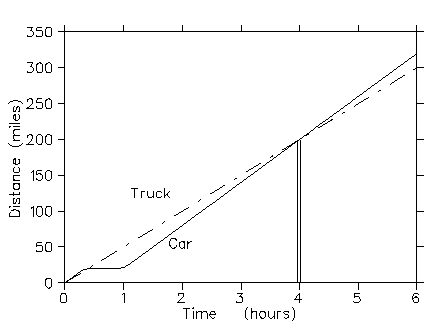
- The two curves intersect at time t=4 hours, i.e. the
car and truck meet again 4 hours after the initial passing.
Problem 15)
- From x = 0.02t3 - 0.1t2 + 2 t cm we get the
speed via
- v = dx/dt = 0.06t2 - 0.2t + 2 cm/s.
- Then
- v1 = 0.06(1)2 - 0.2(1) + 2 = + 1.86 cm/s;
v1 = + 1.86 cm/s
- v5 = 0.06(5)2 - 0.2(5) + 2 = + 2.5 cm/s;
v5 = + 2.5i cm/s
- v10 = 0.06(10)2 - 0.2(10) + 2 = + 6.0 cm/s;
v10 = + 6.0 cm/s
The magnitude of the average velocity is vav = 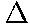 x/
x/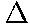 t
= {[0.02(10)3 - 0.1(10)2 + 2(10)]cm} -
{[0.02(0)3 - 0.1(0)2 + 2(0)]cm}/(10s-0s)
= 3.0 cm/s
vav= 3.0 cm/s
The formula is unrealistic for large times because the t2 term in v will produce a ridiculously large
velocity for an ant as t gets large.
t
= {[0.02(10)3 - 0.1(10)2 + 2(10)]cm} -
{[0.02(0)3 - 0.1(0)2 + 2(0)]cm}/(10s-0s)
= 3.0 cm/s
vav= 3.0 cm/s
The formula is unrealistic for large times because the t2 term in v will produce a ridiculously large
velocity for an ant as t gets large.
Problem 17)
- We are told that the acceleration is a constant here, a = 0.6 m/s2
so we have
- v = v0 + at
- Rearranging, we have
- t = (v-v0)/a
- The final velocity is v = 40 mi/hr; we assume that the car starts at rest,
so v0 = 0, and so we have (converting units along the way):
- t = (40 mi/hr)(1 hr/3600 s)(1.609 x 103m/mi)/(0.6 m/s2)
- = 29.8 s
Problem 32)
- For constant acceleration, the average speed is just ½(v0 + v);
thus
- x = vavt = ½(v0+v)t
- x = ½(0 + 4.2 x103 mi/h)(125 s)(1h/3600 s)
- which gives x = 73 mi
Problem 35)
- a)
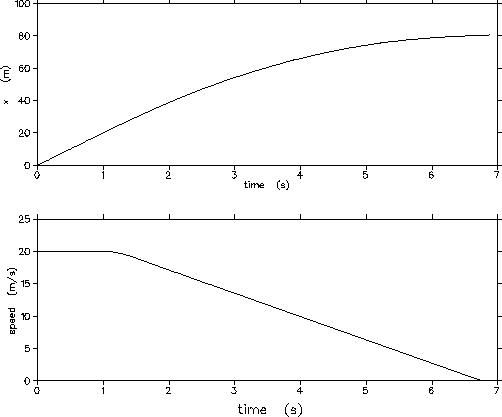
- b) We can first find the distance travelled before the brakes were
applied using
- x1 = v0t1 = (20 m/s)(1.2 s) = 24 m
- We can then find the acceleration while the brakes were applied using
- v2 = v02 + 2 a (x2 - x1)
- thus
- 0 = (20 m/s)2 + 2 a (80 m - 24 m)
- solving for a gives
- a = - 3.57 m/s2
Problem 42)
- With the origin chosen at the point where the brakes are applied, we have
v0 = 40 mi/h = 58.7 ft/s and a = 0.5 g where
g = 32 ft/s2. We can find the distance she travels before stopping from
- v2 = v02 + 2a(x-x0)
- 0 = (58.7 ft/s)2 + 2 [(-0.5(32 ft/s2)](x-0)
- which gives
- x = 107 ft
- Because this is greater than 100 ft, she does not stop before arriving at the light.
Problem 56)
- Let's choose a coordinate system with the origin at the release point,
and with downwards being positive (why should up always be positive?). This is motion
under constant acceleration, so we have
- x = x0 + v0t + (1/2)at2
- Substituting in the values x0 = 0 m, x = 10 m (the ground),
v0 = 0, and a = 25.9 m/s2, we have
- 10 m = 0 + 0 + (1/2)(25.9 m/s2)t2
- which yields
- t = ± 0.88 s
- We take the positive root (can you see why?) to give
- t = 0.88 s
Physics
101 Solutions
Physics 101 Home page
Physics Department Home Page
College of William and Mary,
Dept. of Physics
armd@physics.wm.edu
last updated: August 30 2000


